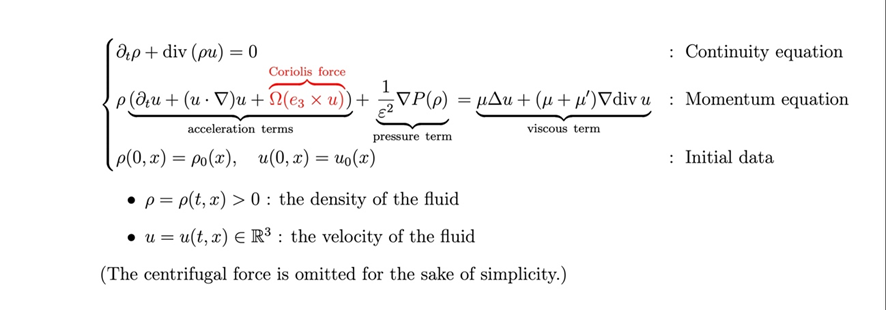
The motion of large-scale geophysical fluids, such as the atmosphere and oceans, has the characteristic property of the Coriolis force due to the effect of the earth’s rotation. In fact, an anisotropic linear term representing the Coriolis force appears in the acceleration terms of the fluid if the Navier-Stokes equations, which are the fundamental equations of the fluid, are rewritten under a rotating coordinate system (Fig.1). “Incompressible rotating fluid”, in which the density of the fluid is constant, is a physically natural situation, and has been the subject of much mathematical research in this case. In particular, since the Coriolis force has a dispersive effect on the linear solution, it is known that a time global solution can be constructed for large data provided that the rotation speed is sufficiently fast, even though the Coriolis force does not affect the energy of the fluid.

(Fig.1)

On the other hand, there seems to be little mathematical research on the case of “compressible rotating fluid” that takes into account changes for the density of the fluid. Although the analysis of the compressibe case has been neglected in geophysical fluid dynamics because the incompressible case is sufficient, compressible rotating flows have important physical applications such as the rotating shallow water equation, and their mathematical analysis is an important issue. My recent research has revealed that compressible rotating fluid have different characteristics from incompressible rotational flows and exhibit characteristic behaviors. The main difference is that the Coriolis force affects the energy of the fluid due to the interaction between the velocity and the gradient of density. In particular, since the Coriolis force is a zero-order linear term, the time-decay rate of the linear solution get worse than the non-rotational case, so there are many difficulties in constructing the time global solvability of the nonlinear solution, but we have solved these difficulties by applying some new technical innovations.

(Fig.2)

In the future work, I will focus on the analysis of the rotating shallow water equation, which is important for applications such as the analysis of Jupiter’s Great Red Spot (Fig.2) . While the compressible rotating Navier-Stokes equation described above is a three-dimensional fluid, the rotating shallow water equation is a two-dimensional flow, which makes it more difficult in the nonlinear estimates of the low frequency parts. In addition, the singular limit of the fast rotation is expected to behave differently from the three-dimensional case, and there are many interesting problems to be solved.